https://www.fightfinance.com

Question 714 return distribution, no explanation

Which of the following quantities is commonly assumed to be normally distributed?

If a variable, say X, is normally distributed with mean ##\mu## and variance ##\sigma^2## then mathematicians write ##X \sim \mathcal{N}(\mu, \sigma^2)##.

If a variable, say Y, is log-normally distributed and the underlying normal distribution has mean ##\mu## and variance ##\sigma^2## then mathematicians write ## Y \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##.

The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue.

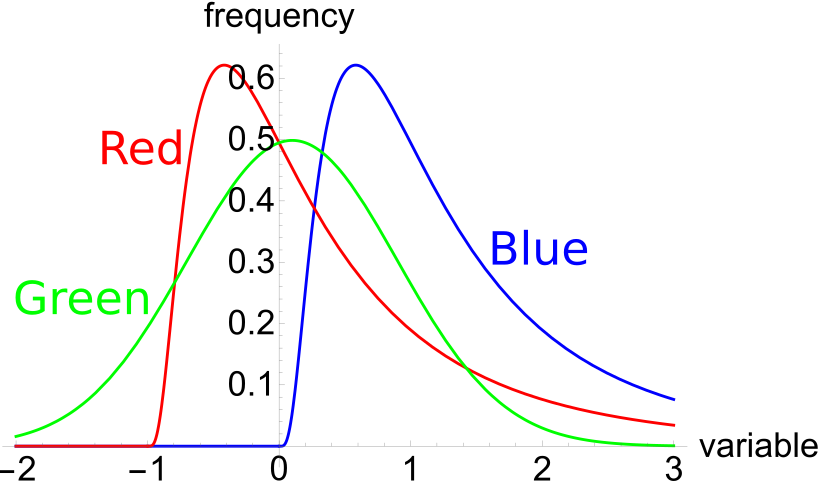
Select the most correct statement:

(a)##Red \sim \mathcal{N}(\mu, \sigma^2)##, ##Green \sim \mathcal{N}(\mu, \sigma^2)## and ##Blue \sim \mathcal{N}(\mu, \sigma^2)##

(b) ##Red \sim \mathcal{N}(\mu, \sigma^2)##, ##Green \sim \mathcal{N}(\mu, \sigma^2)## and ##Blue \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##

(c) ##Red \sim \mathcal{N}(\mu, \sigma^2)##, ##Green \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)## and ##Blue \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##

(d) ##Red \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##, ##Green \sim \mathcal{N}(\mu, \sigma^2)## and ##Blue \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##

(e) ##Red \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##, ##Green \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)## and ##Blue \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##

Question 716 return distribution

The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue.

Which of the below statements is NOT correct?

Question 717 return distribution

The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue. Let ##P_1## be the unknown price of a stock in one year. ##P_1## is a random variable. Let ##P_0 = 1##, so the share price now is $1. This one dollar is a constant, it is not a variable.

Which of the below statements is NOT correct? Financial practitioners commonly assume that the shape of the PDF represented in the colour:

Question 719 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

A stock has an arithmetic average continuously compounded return (AALGDR) of 10% pa, a standard deviation of continuously compounded returns (SDLGDR) of 80% pa and current stock price of $1. Assume that stock prices are log-normally distributed. The graph below summarises this information and provides some helpful formulas.

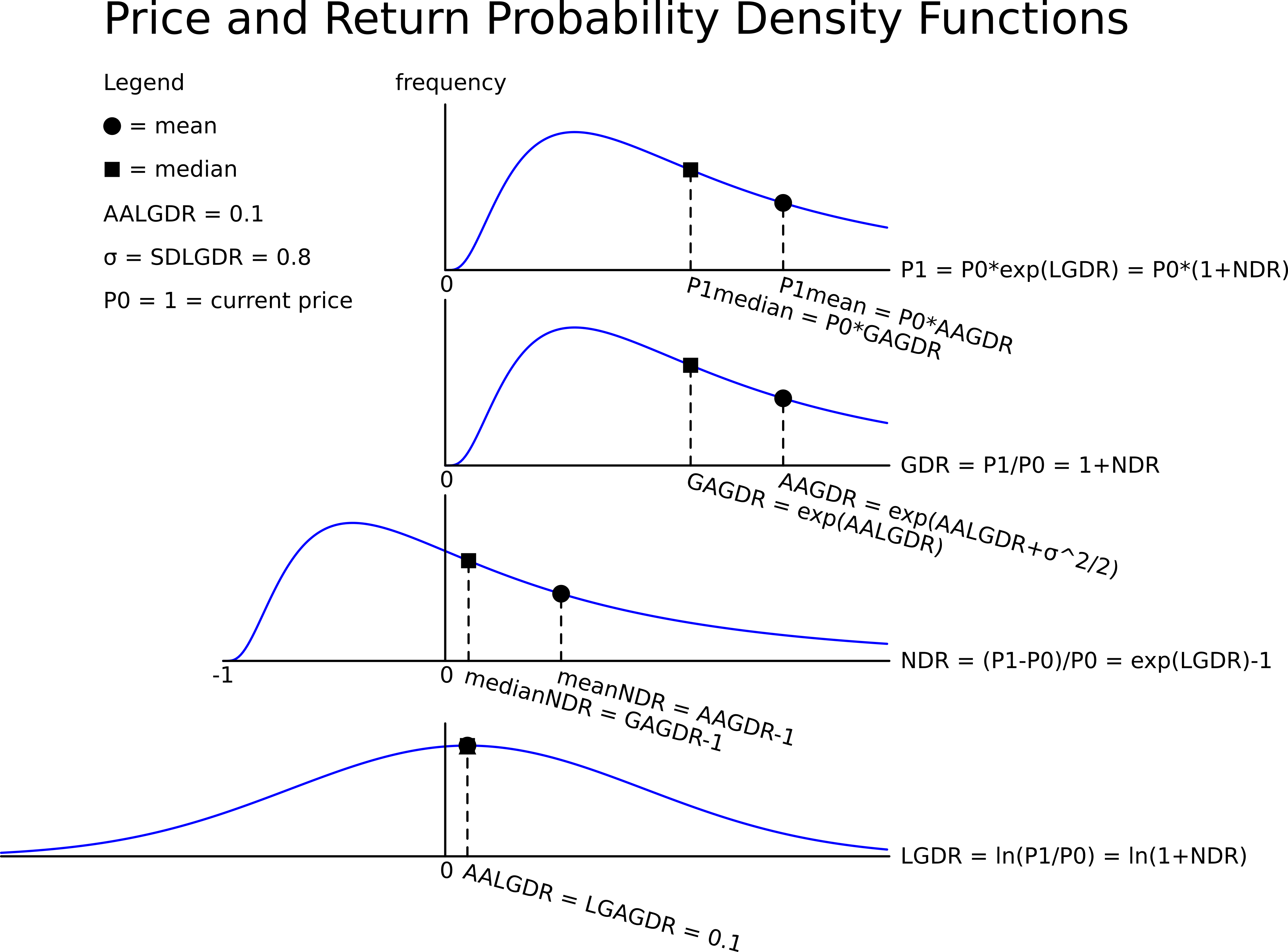
In one year, what do you expect the median and mean prices to be? The answer options are given in the same order.

Question 720 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

In 5 years, what do you expect the median and mean prices to be? The answer options are given in the same order.

Question 721 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

Fred owns some Commonwealth Bank (CBA) shares. He has calculated CBA’s monthly returns for each month in the past 20 years using this formula:

###r_\text{t monthly}=\ln⁡ \left( \dfrac{P_t}{P_{t-1}} \right)###

He then took the arithmetic average and found it to be 1% per month using this formula:

###\bar{r}_\text{monthly}= \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( r_\text{t monthly} \right)} }{T} =0.01=1\% \text{ per month}###

He also found the standard deviation of these monthly returns which was 5% per month:

###\sigma_\text{monthly} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \left( r_\text{t monthly} - \bar{r}_\text{monthly} \right)^2 \right)} }{T} =0.05=5\%\text{ per month}###

Which of the below statements about Fred’s CBA shares is NOT correct? Assume that the past historical average return is the true population average of future expected returns.

(a) The returns ##r_\text{t monthly}## are continuously compounded monthly returns and are likely to be normally distributed, so the mean, median and mode of these continuously compounded returns are all equal to 1% per month.

(b) The annualised average continuously compounded return is ##\bar{r}_\text{cc annual}=12×0.01=0.1=12\%\text{ pa}##. The annualised standard deviation is ##\sigma_\text{annual} = \sqrt{12}×0.05=0.173205081=17.32\%\text{ pa}##.

(c) Over the next 10 years the mean, median and mode continuously compounded 10 year returns will all equal ##0.01 \times 12 \times 10 = 120\%##.

(d) Over the next 10 years the expected mean gross discrete 10 year return is ##e^{(0.01 - 0.05^2/2)×12×10} = 2.857651118## and the median gross discrete 10 year return is ##e^{(0.01 + 0.05^2/2)×12×10}=3.857425531##.

(e) If the current price of the CBA shares is $75, then in 10 years they’re expected to have a mean value of ##75×e^{(0.01 + 0.05^2/2)×12×10} = 289.3069148## and a median value of ##75×e^{0.01×12×10}=249.0087692##.

Question 722 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

Here is a table of stock prices and returns. Which of the statements below the table is NOT correct?

Price and Return Population Statistics
Time	Prices	LGDR	GDR	NDR
0	100
1	50	-0.6931	0.5	-0.5
2	100	0.6931	2	1

Arithmetic average		0	1.25	0.25
Arithmetic standard deviation		0.9802	1.0607	1.0607

(a) The geometric average of the gross discrete returns (GAGDR) equals 1 which is 100%.

(b) ##\text{GAGDR} = \exp \left( \text{AALGDR} \right)##. The GAGDR is equal to the natural exponent of the arithmetic average of the logarithms of the gross discrete returns (AALGDR).

(c) ##\text{GAGDR} = \left( P_T/P_0 \right)^{1/T}##. The GAGDR equals the ratio of the last and first prices raised to the power of the inverse of the number of time periods between them.

(d) ##\text{LGAGDR} = \text{AALGDR}##. This is always true, regardless of the distribution of the prices or returns and the number of return observations. The logarithm of the geometric average of the gross discrete returns (LGAGDR) is always equal to the arithmetic average of the logarithms of the gross discrete returns (AALGDR).

(e) ##\text{LAAGDR} = \text{AALGDR} + \text{SDLGDR}^2/2##. This is always true, regardless of the distribution of the prices or returns and the number of return observations. The logarithm of the arithmetic average of the gross discrete returns (LAAGDR) equals the arithmetic average of the logarithms of the gross discrete returns (AALGDR) plus half the variance of the LGDR's.

Question 723 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

Here is a table of stock prices and returns. Which of the statements below the table is NOT correct?

Price and Return Population Statistics
Time	Prices	LGDR	GDR	NDR
0	100
1	99	-0.010050	0.990000	-0.010000
2	180.40	0.600057	1.822222	0.822222
3	112.73	0.470181	0.624889	0.375111

Arithmetic average		0.0399	1.1457	0.1457
Arithmetic standard deviation		0.4384	0.5011	0.5011

(a) The geometric average of the gross discrete returns (GAGDR) equals 1.04075 which is 104.075%.

(b) ##\exp \left( \text{GAGDR} \right) = \text{AALGDR}##. The natural exponent of the GAGDR equals the arithmetic average of the logarithms of the gross discrete returns (AALGDR).

(c) ##\text{GAGDR}^T = \left( P_T/P_0 \right)##. The GAGDR raised to the power of the number of time period that it's measured over equals the ratio of the first and last prices.

(d) ##\text{GAGDR} = \exp \left( \text{AALGDR} \right)##. This is always true, regardless of the distribution of the prices or returns. The logarithm of the geometric average of the gross discrete returns (LGAGDR) is always equal to the arithmetic average of the logarithm of the gross discrete returns (AALGDR).

(e) ##\text{AAGDR} \approx \exp \left( \text{AALGDR} + \text{SDLGDR}^2/2 \right)##. This is only approximately true and depends on the distribution of the prices and returns. It's asymptotically true as the time period that the returns are measured over reaches infinity.

Question 724 return distribution, mean and median returns

Question 725 return distribution, mean and median returns

Question 726 return distribution, mean and median returns

Question 779 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

Fred owns some BHP shares. He has calculated BHP’s monthly returns for each month in the past 30 years using this formula:

###r_\text{t monthly}=\ln⁡ \left( \dfrac{P_t}{P_{t-1}} \right)###

He then took the arithmetic average and found it to be 0.8% per month using this formula:

###\bar{r}_\text{monthly}= \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( r_\text{t monthly} \right)} }{T} =0.008=0.8\% \text{ per month}###

He also found the standard deviation of these monthly returns which was 15% per month:

###\sigma_\text{monthly} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \left( r_\text{t monthly} - \bar{r}_\text{monthly} \right)^2 \right)} }{T} =0.15=15\%\text{ per month}###

Assume that the past historical average return is the true population average of future expected returns and the stock's returns calculated above ##(r_\text{t monthly})## are normally distributed. Which of the below statements about Fred’s BHP shares is NOT correct?

Question 790 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, log-normal distribution, VaR, confidence interval

A risk manager has identified that their hedge fund’s continuously compounded portfolio returns are normally distributed with a mean of 10% pa and a standard deviation of 30% pa. The hedge fund’s portfolio is currently valued at $100 million. Assume that there is no estimation error in these figures and that the normal cumulative density function at 1.644853627 is 95%.

Which of the following statements is NOT correct? All answers are rounded to the nearest dollar.

Question 791 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, log-normal distribution, VaR, confidence interval

A risk manager has identified that their pension fund’s continuously compounded portfolio returns are normally distributed with a mean of 5% pa and a standard deviation of 20% pa. The fund’s portfolio is currently valued at $1 million. Assume that there is no estimation error in the above figures. To simplify your calculations, all answers below use 2.33 as an approximation for the normal inverse cumulative density function at 99%. All answers are rounded to the nearest dollar. Which of the following statements is NOT correct?

Question 792 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, log-normal distribution, confidence interval

A risk manager has identified that their investment fund’s continuously compounded portfolio returns are normally distributed with a mean of 10% pa and a standard deviation of 40% pa. The fund’s portfolio is currently valued at $1 million. Assume that there is no estimation error in the above figures. To simplify your calculations, all answers below use 2.33 as an approximation for the normal inverse cumulative density function at 99%. All answers are rounded to the nearest dollar. Assume one month is 1/12 of a year. Which of the following statements is NOT correct?

Question 811 log-normal distribution, mean and median returns, return distribution, arithmetic and geometric averages

Which of the following statements about probability distributions is NOT correct?

Question 874 utility, return distribution, log-normal distribution, arithmetic and geometric averages

Who was the first theorist to endorse the maximisiation of the geometric average gross discrete return for investors (not gamblers) since it gave a "...portfolio that has a greater probability of being as valuable or more valuable than any other significantly different portfolio at the end of n years, n being large"?

Question 906 effective rate, return types, net discrete return, return distribution, price gains and returns over time

For an asset's price to double from say $1 to $2 in one year, what must its effective annual return be? Note that an effective annual return is also called a net discrete return per annum. If the price now is ##P_0## and the price in one year is ##P_1## then the effective annul return over the next year is:

###r_\text{effective annual} = \dfrac{P_1 - P_0}{P_0} = \text{NDR}_\text{annual}###

Question 907 continuously compounding rate, return types, return distribution, price gains and returns over time

For an asset's price to double from say $1 to $2 in one year, what must its continuously compounded return ##(r_{CC})## be? If the price now is ##P_0## and the price in one year is ##P_1## then the continuously compounded return over the next year is:

###r_\text{CC annual} = \ln{\left[ \dfrac{P_1}{P_0} \right]} = \text{LGDR}_\text{annual}###

Question 908 effective rate, return types, gross discrete return, return distribution, price gains and returns over time

For an asset's price to double from say $1 to $2 in one year, what must its gross discrete return (GDR) be? If the price now is ##P_0## and the price in one year is ##P_1## then the gross discrete return over the next year is:

###\text{GDR}_\text{annual} = \dfrac{P_1}{P_0}###

Question 921 utility, return distribution, log-normal distribution, arithmetic and geometric averages, no explanation

Who was the first theorist to propose the idea of ‘expected utility’?

Question 925 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, no explanation

The arithmetic average and standard deviation of returns on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 were calculated as follows:

###\bar{r}_\text{yearly} = \dfrac{ \displaystyle\sum\limits_{t=1992}^{24}{\left( \ln⁡ \left( \dfrac{P_{t+1}}{P_t} \right) \right)} }{T} = \text{AALGDR} =0.0949=9.49\% \text{ pa}###

###\sigma_\text{yearly} = \dfrac{ \displaystyle\sum\limits_{t=1992}^{24}{\left( \left( \ln⁡ \left( \dfrac{P_{t+1}}{P_t} \right) - \bar{r}_\text{yearly} \right)^2 \right)} }{T} = \text{SDLGDR} = 0.1692=16.92\text{ pp pa}###

Assume that the log gross discrete returns are normally distributed and that the above estimates are true population statistics, not sample statistics, so there is no standard error in the sample mean or standard deviation estimates. Also assume that the standardised normal Z-statistic corresponding to a one-tail probability of 2.5% is exactly -1.96.

Which of the following statements is NOT correct? If you invested $1m today in the ASX200, then over the next 4 years:

Question 926 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate

The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.

The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.

If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the median dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?

Question 927 mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate

The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.

The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.

If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the mean dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?

Question 928 mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate, no explanation

The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.

The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.

If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the mode dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?

Note that the mode of a log-normally distributed future price is: ##P_{T \text{ mode}} = P_0.e^{(\text{AALGDR} - \text{SDLGDR}^2 ).T} ##

Question 929 standard error, mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate

The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.

The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.

Assume that the data are sample statistics, not population statistics. Assume that the log gross discrete returns are normally distributed.

What is the standard error of your estimate of the sample ASX200 accumulation index arithmetic average log gross discrete return (AALGDR) over the 24 years from 1992 to 2016?

Question 1003 Black-Scholes-Merton option pricing, log-normal distribution, return distribution, hedge fund, risk, financial distress

A hedge fund issued zero coupon bonds with a combined $1 billion face value due to be paid in 3 years. The promised yield to maturity is currently 6% pa given as a continuously compounded return (or log gross discrete return, ##LGDR=\ln[P_T/P_0] \div T##).

The hedge fund owns stock assets worth $1.1 billion now which are expected to have a 10% pa arithmetic average log gross discrete return ##(\text{AALGDR} = \sum\limits_{t=1}^T{\left( \ln[P_t/P_{t-1}] \right)} \div T)## and 30pp pa standard deviation (SDLGDR) in the future.

Analyse the hedge fund using the Merton model of corporate equity as an option on the firm's assets.

The risk free government bond yield to maturity is currently 5% pa given as a continuously compounded return or LGDR.

Which of the below statements is NOT correct? All figures are rounded to the sixth decimal place.

Fight Finance

Courses Tags Random All Recent Scores