Jan asks you for a loan. He wants $100 now and offers to pay you back $120 in 1 year. You can borrow and lend from the bank at an interest rate of 10% pa, given as an effective annual rate.
Ignore credit risk. Remember:
V0=Vt(1+reff)t
Katya offers to pay you $10 at the end of every year for the next 5 years (t=1,2,3,4,5) if you pay her $50 now (t=0). You can borrow and lend from the bank at an interest rate of 10% pa, given as an effective annual rate. Ignore credit risk.
For a price of $129, Joanne will sell you a share which is expected to pay a $30 dividend in one year, and a $10 dividend every year after that forever. So the stock's dividends will be $30 at t=1, $10 at t=2, $10 at t=3, and $10 forever onwards.
The required return of the stock is 10% pa.
Your friend wants to borrow $1,000 and offers to pay you back $100 in 6 months, with more $100 payments at the end of every month for another 11 months. So there will be twelve $100 payments in total. She says that 12 payments of $100 equals $1,200 so she's being generous.
If interest rates are 12% pa, given as an APR compounding monthly, what is the Net Present Value (NPV) of your friend's deal?
Question 22 NPV, perpetuity with growth, effective rate, effective rate conversion
What is the NPV of the following series of cash flows when the discount rate is 10% given as an effective annual rate?
The first payment of $90 is in 3 years, followed by payments every 6 months in perpetuity after that which shrink by 3% every 6 months. That is, the growth rate every 6 months is actually negative 3%, given as an effective 6 month rate. So the payment at t=3.5 years will be 90(1−0.03)1=87.3, and so on.
The required return of a project is 10%, given as an effective annual rate. Assume that the cash flows shown in the table are paid all at once at the given point in time.
What is the Net Present Value (NPV) of the project?
| Project Cash Flows | |
| Time (yrs) | Cash flow ($) |
| 0 | -100 |
| 1 | 0 |
| 2 | 121 |
The phone company Telstra have 2 mobile service plans on offer which both have the same amount of phone call, text message and internet data credit. Both plans have a contract length of 24 months and the monthly cost is payable in advance. The only difference between the two plans is that one is a:
- 'Bring Your Own' (BYO) mobile service plan, costing $50 per month. There is no phone included in this plan. The other plan is a:
- 'Bundled' mobile service plan that comes with the latest smart phone, costing $71 per month. This plan includes the latest smart phone.
Neither plan has any additional payments at the start or end.
The only difference between the plans is the phone, so what is the implied cost of the phone as a present value?
Assume that the discount rate is 2% per month given as an effective monthly rate, the same high interest rate on credit cards.
Question 48 IRR, NPV, bond pricing, premium par and discount bonds, market efficiency
The theory of fixed interest bond pricing is an application of the theory of Net Present Value (NPV). Also, a 'fairly priced' asset is not over- or under-priced. Buying or selling a fairly priced asset has an NPV of zero.
Considering this, which of the following statements is NOT correct?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 0.00 | 1.15 | 1.10 | 1.05 | 1.00 | ... |
After year 4, the annual dividend will grow in perpetuity at -5% pa. Note that this is a negative growth rate, so the dividend will actually shrink. So,
- the dividend at t=5 will be $1(1−0.05)=$0.95,
- the dividend at t=6 will be $1(1−0.05)2=$0.9025, and so on.
The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What is the current price of the stock?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 0.00 | 1.15 | 1.10 | 1.05 | 1.00 | ... |
After year 4, the annual dividend will grow in perpetuity at -5% pa. Note that this is a negative growth rate, so the dividend will actually shrink. So,
- the dividend at t=5 will be $1(1−0.05)=$0.95,
- the dividend at t=6 will be $1(1−0.05)2=$0.9025, and so on.
The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What will be the price of the stock in four and a half years (t = 4.5)?
Question 58 NPV, inflation, real and nominal returns and cash flows, Annuity
A project to build a toll bridge will take two years to complete, costing three payments of $100 million at the start of each year for the next three years, that is at t=0, 1 and 2.
After completion, the toll bridge will yield a constant $50 million at the end of each year for the next 10 years. So the first payment will be at t=3 and the last at t=12. After the last payment at t=12, the bridge will be given to the government.
The required return of the project is 21% pa given as an effective annual nominal rate.
All cash flows are real and the expected inflation rate is 10% pa given as an effective annual rate. Ignore taxes.
The Net Present Value is:
The required return of a project is 10%, given as an effective annual rate. Assume that the cash flows shown in the table are paid all at once at the given point in time.
What is the Net Present Value (NPV) of the project?
| Project Cash Flows | |
| Time (yrs) | Cash flow ($) |
| 0 | -100 |
| 1 | 11 |
| 2 | 121 |
In Australia, domestic university students are allowed to buy concession tickets for the bus, train and ferry which sell at a discount of 50% to full-price tickets.
The Australian Government do not allow international university students to buy concession tickets, they have to pay the full price.
Some international students see this as unfair and they are willing to pay for fake university identification cards which have the concession sticker.
What is the most that an international student would be willing to pay for a fake identification card?
Assume that international students:
- consider buying their fake card on the morning of the first day of university from their neighbour, just before they leave to take the train into university.
- buy their weekly train tickets on the morning of the first day of each week.
- ride the train to university and back home again every day seven days per week until summer holidays 40 weeks from now. The concession card only lasts for those 40 weeks. Assume that there are 52 weeks in the year for the purpose of interest rate conversion.
- a single full-priced one-way train ride costs $5.
- have a discount rate of 11% pa, given as an effective annual rate.
Approach this question from a purely financial view point, ignoring the illegality, embarrassment and the morality of committing fraud.
The theory of fixed interest bond pricing is an application of the theory of Net Present Value (NPV). Also, a 'fairly priced' asset is not over- or under-priced. Buying or selling a fairly priced asset has an NPV of zero.
Considering this, which of the following statements is NOT correct?
A person is thinking about borrowing $100 from the bank at 7% pa and investing it in shares with an expected return of 10% pa. One year later the person intends to sell the shares and pay back the loan in full. Both the loan and the shares are fairly priced.
What is the Net Present Value (NPV) of this one year investment? Note that you are asked to find the present value (V0), not the value in one year (V1).
The security market line (SML) shows the relationship between beta and expected return.
Buying investment projects that plot above the SML would lead to:
The following cash flows are expected:
- 10 yearly payments of $60, with the first payment in 3 years from now (first payment at t=3 and last at t=12).
- 1 payment of $400 in 5 years and 6 months (t=5.5) from now.
What is the NPV of the cash flows if the discount rate is 10% given as an effective annual rate?
A project has an internal rate of return (IRR) which is greater than its required return. Select the most correct statement.
A text book publisher is thinking of asking some teachers to write a new textbook at a cost of $100,000, payable now. The book would be written, printed and ready to sell to students in 2 years. It will be ready just before semester begins.
A cash flow of $100 would be made from each book sold, after all costs such as printing and delivery. There are 600 students per semester. Assume that every student buys a new text book. Remember that there are 2 semesters per year and students buy text books at the beginning of the semester.
Assume that text book publishers will sell the books at the same price forever and that the number of students is constant.
If the discount rate is 8% pa, given as an effective annual rate, what is the NPV of the project?
A student just won the lottery. She won $1 million in cash after tax. She is trying to calculate how much she can spend per month for the rest of her life. She assumes that she will live for another 60 years. She wants to withdraw equal amounts at the beginning of every month, starting right now.
All of the cash is currently sitting in a bank account which pays interest at a rate of 6% pa, given as an APR compounding per month. On her last withdrawal, she intends to have nothing left in her bank account. How much can she withdraw at the beginning of each month?
The following cash flows are expected:
- 10 yearly payments of $80, with the first payment in 3 years from now (first payment at t=3).
- 1 payment of $600 in 5 years and 6 months (t=5.5) from now.
What is the NPV of the cash flows if the discount rate is 10% given as an effective annual rate?
A project's net present value (NPV) is negative. Select the most correct statement.
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 8 | 8 | 8 | 20 | 8 | ... |
After year 4, the dividend will grow in perpetuity at 4% pa. The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates. Note that the $8 dividend at time zero is about to be paid tonight.
What is the current price of the stock?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 8 | 8 | 8 | 20 | 8 | ... |
After year 4, the dividend will grow in perpetuity at 4% pa. The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates. Note that the $8 dividend at time zero is about to be paid tonight.
What will be the price of the stock in 5 years (t = 5), just after the dividend at that time has been paid?
A project's NPV is positive. Select the most correct statement:
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 2 | 2 | 2 | 10 | 3 | ... |
After year 4, the dividend will grow in perpetuity at 4% pa. The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What is the current price of the stock?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 2 | 2 | 2 | 10 | 3 | ... |
After year 4, the dividend will grow in perpetuity at 4% pa. The required return on the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What will be the price of the stock in 5 years (t = 5), just after the dividend at that time has been paid?
A project's Profitability Index (PI) is less than 1. Select the most correct statement:
Harvey Norman the large retailer often runs sales advertising 2 years interest free when you purchase its products. This offer can be seen as a free personal loan from Harvey Norman to its customers.
Assume that banks charge an interest rate on personal loans of 12% pa given as an APR compounding per month. This is the interest rate that Harvey Norman deserves on the 2 year loan it extends to its customers. Therefore Harvey Norman must implicitly include the cost of this loan in the advertised sale price of its goods.
If you were a customer buying from Harvey Norman, and you were paying immediately, not in 2 years, what is the minimum percentage discount to the advertised sale price that you would insist on? (Hint: if it makes it easier, assume that you’re buying a product with an advertised price of $100).
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 0 | 6 | 12 | 18 | 20 | ... |
After year 4, the dividend will grow in perpetuity at 5% pa. The required return of the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What is the current price of the stock?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 0 | 6 | 12 | 18 | 20 | ... |
After year 4, the dividend will grow in perpetuity at 5% pa. The required return of the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
What will be the price of the stock in 7 years (t = 7), just after the dividend at that time has been paid?
A stock is expected to pay the following dividends:
| Cash Flows of a Stock | ||||||
| Time (yrs) | 0 | 1 | 2 | 3 | 4 | ... |
| Dividend ($) | 0 | 6 | 12 | 18 | 20 | ... |
After year 4, the dividend will grow in perpetuity at 5% pa. The required return of the stock is 10% pa. Both the growth rate and required return are given as effective annual rates.
If all of the dividends since time period zero were deposited into a bank account yielding 8% pa as an effective annual rate, how much money will be in the bank account in 2.5 years (in other words, at t=2.5)?
A stock is expected to pay a dividend of $15 in one year (t=1), then $25 for 9 years after that (payments at t=2 ,3,...10), and on the 11th year (t=11) the dividend will be 2% less than at t=10, and will continue to shrink at the same rate every year after that forever. The required return of the stock is 10%. All rates are effective annual rates.
What is the price of the stock now?
Question 218 NPV, IRR, profitability index, average accounting return
Which of the following statements is NOT correct?
A very low-risk stock just paid its semi-annual dividend of $0.14, as it has for the last 5 years. You conservatively estimate that from now on the dividend will fall at a rate of 1% every 6 months.
If the stock currently sells for $3 per share, what must be its required total return as an effective annual rate?
If risk free government bonds are trading at a yield of 4% pa, given as an effective annual rate, would you consider buying or selling the stock?
The stock's required total return is:
Question 235 SML, NPV, CAPM, risk
The security market line (SML) shows the relationship between beta and expected return.
Investment projects that plot on the SML would have:
Question 241 Miller and Modigliani, leverage, payout policy, diversification, NPV
One of Miller and Modigliani's (M&M's) important insights is that a firm's managers should not try to achieve a particular level of leverage in a world with zero taxes and perfect information since investors can make their own leverage. Therefore corporate capital structure policy is irrelevant since investors can achieve their own desired leverage at the personal level by borrowing or lending on their own.
This principal of 'home-made' or 'do-it-yourself' leverage can also be applied to other topics. Read the following statements to decide which are true:
(I) Payout policy: a firm's managers should not try to achieve a particular pattern of equity payout.
(II) Agency costs: a firm's managers should not try to minimise agency costs.
(III) Diversification: a firm's managers should not try to diversify across industries.
(IV) Shareholder wealth: a firm's managers should not try to maximise shareholders' wealth.
Which of the above statement(s) are true?
Question 244 CAPM, SML, NPV, risk
Examine the following graph which shows stocks' betas (β) and expected returns (μ):
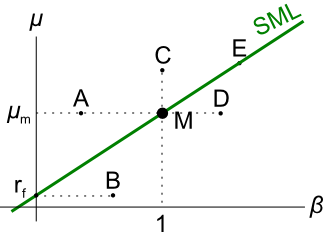
Assume that the CAPM holds and that future expectations of stocks' returns and betas are correctly measured. Which statement is NOT correct?
Your neighbour asks you for a loan of $100 and offers to pay you back $120 in one year.
You don't actually have any money right now, but you can borrow and lend from the bank at a rate of 10% pa. Rates are given as effective annual rates.
Assume that your neighbour will definitely pay you back. Ignore interest tax shields and transaction costs.
The Net Present Value (NPV) of lending to your neighbour is $9.09. Describe what you would do to actually receive a $9.09 cash flow right now with zero net cash flows in the future.
You have $100,000 in the bank. The bank pays interest at 10% pa, given as an effective annual rate.
You wish to consume an equal amount now (t=0) and in one year (t=1) and have nothing left in the bank at the end (t=1).
How much can you consume at each time?
You have $100,000 in the bank. The bank pays interest at 10% pa, given as an effective annual rate.
You wish to consume an equal amount now (t=0), in one year (t=1) and in two years (t=2), and still have $50,000 in the bank after that (t=2).
How much can you consume at each time?
You just started work at your new job which pays $48,000 per year.
The human resources department have given you the option of being paid at the end of every week or every month.
Assume that there are 4 weeks per month, 12 months per year and 48 weeks per year.
Bank interest rates are 12% pa given as an APR compounding per month.
What is the dollar gain over one year, as a net present value, of being paid every week rather than every month?
Suppose you had $100 in a savings account and the interest rate was 2% per year.
After 5 years, how much do you think you would have in the account if you left the money to grow?
Question 295 inflation, real and nominal returns and cash flows, NPV
When valuing assets using discounted cash flow (net present value) methods, it is important to consider inflation. To properly deal with inflation:
(I) Discount nominal cash flows by nominal discount rates.
(II) Discount nominal cash flows by real discount rates.
(III) Discount real cash flows by nominal discount rates.
(IV) Discount real cash flows by real discount rates.
Which of the above statements is or are correct?
What is the net present value (NPV) of undertaking a full-time Australian undergraduate business degree as an Australian citizen? Only include the cash flows over the duration of the degree, ignore any benefits or costs of the degree after it's completed.
Assume the following:
- The degree takes 3 years to complete and all students pass all subjects.
- There are 2 semesters per year and 4 subjects per semester.
- University fees per subject per semester are $1,277, paid at the start of each semester. Fees are expected to remain constant in real terms for the next 3 years.
- There are 52 weeks per year.
- The first semester is just about to start (t=0). The first semester lasts for 19 weeks (t=0 to 19).
- The second semester starts immediately afterwards (t=19) and lasts for another 19 weeks (t=19 to 38).
- The summer holidays begin after the second semester ends and last for 14 weeks (t=38 to 52). Then the first semester begins the next year, and so on.
- Working full time at the grocery store instead of studying full-time pays $20/hr and you can work 35 hours per week. Wages are paid at the end of each week and are expected to remain constant in real terms.
- Full-time students can work full-time during the summer holiday at the grocery store for the same rate of $20/hr for 35 hours per week.
- The discount rate is 9.8% pa. All rates and cash flows are real. Inflation is expected to be 3% pa. All rates are effective annual.
The NPV of costs from undertaking the university degree is:
Question 345 capital budgeting, break even, NPV
| Project Data | ||
| Project life | 10 yrs | |
| Initial investment in factory | $10m | |
| Depreciation of factory per year | $1m | |
| Expected scrap value of factory at end of project | $0 | |
| Sale price per unit | $10 | |
| Variable cost per unit | $6 | |
| Fixed costs per year, paid at the end of each year | $2m | |
| Interest expense per year | 0 | |
| Tax rate | 30% | |
| Cost of capital per annum | 10% | |
Notes
- The firm's current liabilities are forecast to stay at $0.5m. The firm's current assets (mostly inventory) is currently $1m, but is forecast to grow by $0.1m at the end of each year due to the project.
At the end of the project, the current assets accumulated due to the project can be sold for the same price that they were bought. - A marketing survey was used to forecast sales. It cost $1.4m which was just paid. The cost has been capitalised by the accountants and is tax-deductible over the life of the project, regardless of whether the project goes ahead or not. This amortisation expense is not included in the depreciation expense listed in the table above.
Assumptions
- All cash flows occur at the start or end of the year as appropriate, not in the middle or throughout the year.
- All rates and cash flows are real. The inflation rate is 3% pa.
- All rates are given as effective annual rates.
Find the break even unit production (Q) per year to achieve a zero Net Income (NI) and Net Present Value (NPV), respectively. The answers below are listed in the same order.
Your poor friend asks to borrow some money from you. He would like $1,000 now (t=0) and every year for the next 5 years, so there will be 6 payments of $1,000 from t=0 to t=5 inclusive. In return he will pay you $10,000 in seven years from now (t=7).
What is the net present value (NPV) of lending to your friend?
Assume that your friend will definitely pay you back so the loan is risk-free, and that the yield on risk-free government debt is 10% pa, given as an effective annual rate.
Your friend overheard that you need some cash and asks if you would like to borrow some money. She can lend you $5,000 now (t=0), and in return she wants you to pay her back $1,000 in two years (t=2) and every year after that for the next 5 years, so there will be 6 payments of $1,000 from t=2 to t=7 inclusive.
What is the net present value (NPV) of borrowing from your friend?
Assume that banks loan funds at interest rates of 10% pa, given as an effective annual rate.
Your friend is trying to find the net present value of an investment which:
- Costs $1 million initially (t=0); and
- Pays a single positive cash flow of $1.1 million in one year (t=1).
The investment has a total required return of 10% pa due to its moderate level of undiversifiable risk.
Your friend is aware of the importance of opportunity costs and the time value of money, but he is unsure of how to find the NPV of the project.
He knows that the opportunity cost of investing the $1m in the project is the expected gain from investing the money in shares instead. Like the project, shares also have an expected return of 10% since they have moderate undiversifiable risk. This opportunity cost is $0.1m (=1m×10%) which occurs in one year (t=1).
He knows that the time value of money should be accounted for, and this can be done by finding the present value of the cash flows in one year.
Your friend has listed a few different ways to find the NPV which are written down below.
Method 1: −1m+1.1m(1+0.1)1
Method 2: −1m+1.1m−1m×0.1
Method 3: −1m+1.1m(1+0.1)1−1m×0.1
Which of the above calculations give the correct NPV? Select the most correct answer.
Question 370 capital budgeting, NPV, interest tax shield, WACC, CFFA
| Project Data | ||
| Project life | 2 yrs | |
| Initial investment in equipment | $600k | |
| Depreciation of equipment per year | $250k | |
| Expected sale price of equipment at end of project | $200k | |
| Revenue per job | $12k | |
| Variable cost per job | $4k | |
| Quantity of jobs per year | 120 | |
| Fixed costs per year, paid at the end of each year | $100k | |
| Interest expense in first year (at t=1) | $16.091k | |
| Interest expense in second year (at t=2) | $9.711k | |
| Tax rate | 30% | |
| Government treasury bond yield | 5% | |
| Bank loan debt yield | 6% | |
| Levered cost of equity | 12.5% | |
| Market portfolio return | 10% | |
| Beta of assets | 1.24 | |
| Beta of levered equity | 1.5 | |
| Firm's and project's debt-to-equity ratio | 25% | |
Notes
- The project will require an immediate purchase of $50k of inventory, which will all be sold at cost when the project ends. Current liabilities are negligible so they can be ignored.
Assumptions
- The debt-to-equity ratio will be kept constant throughout the life of the project. The amount of interest expense at the end of each period has been correctly calculated to maintain this constant debt-to-equity ratio. Note that interest expense is different in each year.
- Thousands are represented by 'k' (kilo).
- All cash flows occur at the start or end of the year as appropriate, not in the middle or throughout the year.
- All rates and cash flows are nominal. The inflation rate is 2% pa.
- All rates are given as effective annual rates.
- The 50% capital gains tax discount is not available since the project is undertaken by a firm, not an individual.
What is the net present value (NPV) of the project?
Question 397 financial distress, leverage, capital structure, NPV
A levered firm has a market value of assets of $10m. Its debt is all comprised of zero-coupon bonds which mature in one year and have a combined face value of $9.9m.
Investors are risk-neutral and therefore all debt and equity holders demand the same required return of 10% pa.
Therefore the current market capitalisation of debt (D0) is $9m and equity (E0) is $1m.
A new project presents itself which requires an investment of $2m and will provide a:
- $6.6m cash flow with probability 0.5 in the good state of the world, and a
- -$4.4m (notice the negative sign) cash flow with probability 0.5 in the bad state of the world.
The project can be funded using the company's excess cash, no debt or equity raisings are required.
What would be the new market capitalisation of equity (E0, with project) if shareholders vote to proceed with the project, and therefore should shareholders proceed with the project?
Question 398 financial distress, capital raising, leverage, capital structure, NPV
A levered firm has zero-coupon bonds which mature in one year and have a combined face value of $9.9m.
Investors are risk-neutral and therefore all debt and equity holders demand the same required return of 10% pa.
In one year the firm's assets will be worth:
- $13.2m with probability 0.5 in the good state of the world, or
- $6.6m with probability 0.5 in the bad state of the world.
A new project presents itself which requires an investment of $2m and will provide a certain cash flow of $3.3m in one year.
The firm doesn't have any excess cash to make the initial $2m investment, but the funds can be raised from shareholders through a fairly priced rights issue. Ignore all transaction costs.
Should shareholders vote to proceed with the project and equity raising? What will be the gain in shareholder wealth if they decide to proceed?
A pharmaceutical firm has just discovered a valuable new drug. So far the news has been kept a secret.
The net present value of making and commercialising the drug is $200 million, but $600 million of bonds will need to be issued to fund the project and buy the necessary plant and equipment.
The firm will release the news of the discovery and bond raising to shareholders simultaneously in the same announcement. The bonds will be issued shortly after.
Once the announcement is made and the bonds are issued, what is the expected increase in the value of the firm's assets (ΔV), market capitalisation of debt (ΔD) and market cap of equity (ΔE)?
The triangle symbol is the Greek letter capital delta which means change or increase in mathematics.
Ignore the benefit of interest tax shields from having more debt.
Remember: ΔV = ΔD+ΔE
A managed fund charges fees based on the amount of money that you keep with them. The fee is 2% of the end-of-year amount, paid at the end of every year.
This fee is charged regardless of whether the fund makes gains or losses on your money.
The fund offers to invest your money in shares which have an expected return of 10% pa before fees.
You are thinking of investing $100,000 in the fund and keeping it there for 40 years when you plan to retire.
How much money do you expect to have in the fund in 40 years? Also, what is the future value of the fees that the fund expects to earn from you? Give both amounts as future values in 40 years. Assume that:
- The fund has no private information.
- Markets are weak and semi-strong form efficient.
- The fund's transaction costs are negligible.
- The cost and trouble of investing your money in shares by yourself, without the managed fund, is negligible.
- The fund invests its fees in the same companies as it invests your funds in, but with no fees.
The below answer choices list your expected wealth in 40 years, and then the fund's expected wealth in 40 years.
Question 418 capital budgeting, NPV, interest tax shield, WACC, CFFA, CAPM
| Project Data | ||
| Project life | 1 year | |
| Initial investment in equipment | $8m | |
| Depreciation of equipment per year | $8m | |
| Expected sale price of equipment at end of project | 0 | |
| Unit sales per year | 4m | |
| Sale price per unit | $10 | |
| Variable cost per unit | $5 | |
| Fixed costs per year, paid at the end of each year | $2m | |
| Interest expense in first year (at t=1) | $0.562m | |
| Corporate tax rate | 30% | |
| Government treasury bond yield | 5% | |
| Bank loan debt yield | 9% | |
| Market portfolio return | 10% | |
| Covariance of levered equity returns with market | 0.32 | |
| Variance of market portfolio returns | 0.16 | |
| Firm's and project's debt-to-equity ratio | 50% | |
Notes
- Due to the project, current assets will increase by $6m now (t=0) and fall by $6m at the end (t=1). Current liabilities will not be affected.
Assumptions
- The debt-to-equity ratio will be kept constant throughout the life of the project. The amount of interest expense at the end of each period has been correctly calculated to maintain this constant debt-to-equity ratio.
- Millions are represented by 'm'.
- All cash flows occur at the start or end of the year as appropriate, not in the middle or throughout the year.
- All rates and cash flows are real. The inflation rate is 2% pa. All rates are given as effective annual rates.
- The project is undertaken by a firm, not an individual.
What is the net present value (NPV) of the project?
Question 419 capital budgeting, NPV, interest tax shield, WACC, CFFA, CAPM, no explanation
| Project Data | ||
| Project life | 1 year | |
| Initial investment in equipment | $6m | |
| Depreciation of equipment per year | $6m | |
| Expected sale price of equipment at end of project | 0 | |
| Unit sales per year | 9m | |
| Sale price per unit | $8 | |
| Variable cost per unit | $6 | |
| Fixed costs per year, paid at the end of each year | $1m | |
| Interest expense in first year (at t=1) | $0.53m | |
| Tax rate | 30% | |
| Government treasury bond yield | 5% | |
| Bank loan debt yield | 6% | |
| Market portfolio return | 10% | |
| Covariance of levered equity returns with market | 0.08 | |
| Variance of market portfolio returns | 0.16 | |
| Firm's and project's debt-to-assets ratio | 50% | |
Notes
- Due to the project, current assets will increase by $5m now (t=0) and fall by $5m at the end (t=1). Current liabilities will not be affected.
Assumptions
- The debt-to-assets ratio will be kept constant throughout the life of the project. The amount of interest expense at the end of each period has been correctly calculated to maintain this constant debt-to-equity ratio.
- Millions are represented by 'm'.
- All cash flows occur at the start or end of the year as appropriate, not in the middle or throughout the year.
- All rates and cash flows are real. The inflation rate is 2% pa.
- All rates are given as effective annual rates.
- The 50% capital gains tax discount is not available since the project is undertaken by a firm, not an individual.
What is the net present value (NPV) of the project?
A mining firm has just discovered a new mine. So far the news has been kept a secret.
The net present value of digging the mine and selling the minerals is $250 million, but $500 million of new equity and $300 million of new bonds will need to be issued to fund the project and buy the necessary plant and equipment.
The firm will release the news of the discovery and equity and bond raising to shareholders simultaneously in the same announcement. The shares and bonds will be issued shortly after.
Once the announcement is made and the new shares and bonds are issued, what is the expected increase in the value of the firm's assets (\Delta V), market capitalisation of debt (\Delta D) and market cap of equity (\Delta E)? Assume that markets are semi-strong form efficient.
The triangle symbol \Delta is the Greek letter capital delta which means change or increase in mathematics.
Ignore the benefit of interest tax shields from having more debt.
Remember: \Delta V = \Delta D+ \Delta E
A company advertises an investment costing $1,000 which they say is underpriced. They say that it has an expected total return of 15% pa, but a required return of only 10% pa. Assume that there are no dividend payments so the entire 15% total return is all capital return.
Assuming that the company's statements are correct, what is the NPV of buying the investment if the 15% return lasts for the next 100 years (t=0 to 100), then reverts to 10% pa after that time? Also, what is the NPV of the investment if the 15% return lasts forever?
In both cases, assume that the required return of 10% remains constant. All returns are given as effective annual rates.
The answer choices below are given in the same order (15% for 100 years, and 15% forever):
The boss of WorkingForTheManCorp has a wicked (and unethical) idea. He plans to pay his poor workers one week late so that he can get more interest on his cash in the bank.
Every week he is supposed to pay his 1,000 employees $1,000 each. So $1 million is paid to employees every week.
The boss was just about to pay his employees today, until he thought of this idea so he will actually pay them one week (7 days) later for the work they did last week and every week in the future, forever.
Bank interest rates are 10% pa, given as a real effective annual rate. So r_\text{eff annual, real} = 0.1 and the real effective weekly rate is therefore r_\text{eff weekly, real} = (1+0.1)^{1/52}-1 = 0.001834569
All rates and cash flows are real, the inflation rate is 3% pa and there are 52 weeks per year. The boss will always pay wages one week late. The business will operate forever with constant real wages and the same number of employees.
What is the net present value (NPV) of the boss's decision to pay later?
Discounted cash flow (DCF) valuation prices assets by finding the present value of the asset's future cash flows. The single cash flow, annuity, and perpetuity equations are very useful for this.
Which of the following equations is the 'perpetuity with growth' equation?
What type of present value equation is best suited to value a residential house investment property that is expected to pay constant rental payments forever? Note that 'constant' has the same meaning as 'level' in this context.
A firm is considering a business project which costs $11m now and is expected to pay a constant $1m at the end of every year forever.
Assume that the initial $11m cost is funded using the firm's existing cash so no new equity or debt will be raised. The cost of capital is 10% pa.
Which of the following statements about net present value (NPV), internal rate of return (IRR) and payback period is NOT correct?
A firm is considering a business project which costs $10m now and is expected to pay a single cash flow of $12.1m in two years.
Assume that the initial $10m cost is funded using the firm's existing cash so no new equity or debt will be raised. The cost of capital is 10% pa.
Which of the following statements about net present value (NPV), internal rate of return (IRR) and payback period is NOT correct?
Question 498 NPV, Annuity, perpetuity with growth, multi stage growth model
A business project is expected to cost $100 now (t=0), then pay $10 at the end of the third (t=3), fourth, fifth and sixth years, and then grow by 5% pa every year forever. So the cash flow will be $10.5 at the end of the seventh year (t=7), then $11.025 at the end of the eighth year (t=8) and so on perpetually. The total required return is 10℅ pa.
Which of the following formulas will NOT give the correct net present value of the project?
Some countries' interest rates are so low that they're zero.
If interest rates are 0% pa and are expected to stay at that level for the foreseeable future, what is the most that you would be prepared to pay a bank now if it offered to pay you $10 at the end of every year for the next 5 years?
In other words, what is the present value of five $10 payments at time 1, 2, 3, 4 and 5 if interest rates are 0% pa?
The below graph shows a project's net present value (NPV) against its annual discount rate.
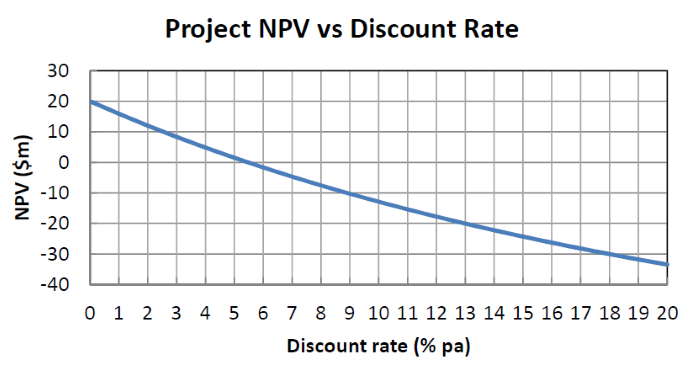
For what discount rate or range of discount rates would you accept and commence the project?
All answer choices are given as approximations from reading off the graph.
The below graph shows a project's net present value (NPV) against its annual discount rate.

Which of the following statements is NOT correct?
An investor owns an empty block of land that has local government approval to be developed into a petrol station, car wash or car park. The council will only allow a single development so the projects are mutually exclusive.
All of the development projects have the same risk and the required return of each is 10% pa. Each project has an immediate cost and once construction is finished in one year the land and development will be sold. The table below shows the estimated costs payable now, expected sale prices in one year and the internal rates of returns (IRR's).
| Mutually Exclusive Projects | |||
| Project | Cost now ($) |
Sale price in one year ($) |
IRR (% pa) |
| Petrol station | 9,000,000 | 11,000,000 | 22.22 |
| Car wash | 800,000 | 1,100,000 | 37.50 |
| Car park | 70,000 | 110,000 | 57.14 |
Which project should the investor accept?
A share currently worth $100 is expected to pay a constant dividend of $4 for the next 5 years with the first dividend in one year (t=1) and the last in 5 years (t=5).
The total required return is 10% pa.
What do you expected the share price to be in 5 years, just after the dividend at that time has been paid?
The following cash flows are expected:
- Constant perpetual yearly payments of $70, with the first payment in 2.5 years from now (first payment at t=2.5).
- A single payment of $600 in 3 years and 9 months (t=3.75) from now.
What is the NPV of the cash flows if the discount rate is 10% given as an effective annual rate?
The following cash flows are expected:
- 10 yearly payments of $80, with the first payment in 6.5 years from now (first payment at t=6.5).
- A single payment of $500 in 4 years and 3 months (t=4.25) from now.
What is the NPV of the cash flows if the discount rate is 10% given as an effective annual rate?
An investor owns a whole level of an old office building which is currently worth $1 million. There are three mutually exclusive projects that can be started by the investor. The office building level can be:
- Rented out to a tenant for one year at $0.1m paid immediately, and then sold for $0.99m in one year.
- Refurbished into more modern commercial office rooms at a cost of $1m now, and then sold for $2.4m when the refurbishment is finished in one year.
- Converted into residential apartments at a cost of $2m now, and then sold for $3.4m when the conversion is finished in one year.
All of the development projects have the same risk so the required return of each is 10% pa. The table below shows the estimated cash flows and internal rates of returns (IRR's).
| Mutually Exclusive Projects | |||
| Project | Cash flow now ($) |
Cash flow in one year ($) |
IRR (% pa) |
| Rent then sell as is | -900,000 | 990,000 | 10 |
| Refurbishment into modern offices | -2,000,000 | 2,400,000 | 20 |
| Conversion into residential apartments | -3,000,000 | 3,400,000 | 13.33 |
Which project should the investor accept?
You have $100,000 in the bank. The bank pays interest at 10% pa, given as an effective annual rate.
You wish to consume twice as much now (t=0) as in one year (t=1) and have nothing left in the bank at the end.
How much can you consume at time zero and one? The answer choices are given in the same order.
You have $100,000 in the bank. The bank pays interest at 10% pa, given as an effective annual rate.
You wish to consume half as much now (t=0) as in one year (t=1) and have nothing left in the bank at the end.
How much can you consume at time zero and one? The answer choices are given in the same order.
Question 542 price gains and returns over time, IRR, NPV, income and capital returns, effective return
For an asset price to double every 10 years, what must be the expected future capital return, given as an effective annual rate?
Question 543 price gains and returns over time, IRR, NPV, income and capital returns, effective return
For an asset price to triple every 5 years, what must be the expected future capital return, given as an effective annual rate?
Question 574 inflation, real and nominal returns and cash flows, NPV
What is the present value of a nominal payment of $100 in 5 years? The real discount rate is 10% pa and the inflation rate is 3% pa.

Assets A, B, M and r_f are shown on the graphs above. Asset M is the market portfolio and r_f is the risk free yield on government bonds. Which of the below statements is NOT correct?
A company advertises an investment costing $1,000 which they say is underpriced. They say that it has an expected total return of 15% pa, but a required return of only 10% pa. Of the 15% pa total expected return, the dividend yield is expected to always be 7% pa and rest is the capital yield.
Assuming that the company's statements are correct, what is the NPV of buying the investment if the 15% total return lasts for the next 100 years (t=0 to 100), then reverts to 10% after that time? Also, what is the NPV of the investment if the 15% return lasts forever?
In both cases, assume that the required return of 10% remains constant, the dividends can only be re-invested at 10% pa and all returns are given as effective annual rates.
The answer choices below are given in the same order (15% for 100 years, and 15% forever):
Question 749 Multiples valuation, PE ratio, price to revenue ratio, price to book ratio, NPV
A real estate agent says that the price of a house in Sydney Australia is approximately equal to the gross weekly rent times 1000.
What type of valuation method is the real estate agent using?
Telsa Motors advertises that its Model S electric car saves $570 per month in fuel costs. Assume that Tesla cars last for 10 years, fuel and electricity costs remain the same, and savings are made at the end of each month with the first saving of $570 in one month from now.
The effective annual interest rate is 15.8%, and the effective monthly interest rate is 1.23%. What is the present value of the savings?
The following cash flows are expected:
- A perpetuity of yearly payments of $30, with the first payment in 5 years (first payment at t=5, which continues every year after that forever).
- One payment of $100 in 6 years and 3 months (t=6.25).
What is the NPV of the cash flows if the discount rate is 10% given as an effective annual rate?
The phone company Optus have 2 mobile service plans on offer which both have the same amount of phone call, text message and internet data credit. Both plans have a contract length of 24 months and the monthly cost is payable in advance. The only difference between the two plans is that one is a:
- 'Bring Your Own' (BYO) mobile service plan, costing $80 per month. There is no phone included in this plan. The other plan is a:
- 'Bundled' mobile service plan that comes with the latest smart phone, costing $100 per month. This plan includes the latest smart phone.
Neither plan has any additional payments at the start or end. Assume that the discount rate is 1% per month given as an effective monthly rate.
The only difference between the plans is the phone, so what is the implied cost of the phone as a present value? Given that the latest smart phone actually costs $600 to purchase outright from another retailer, should you commit to the BYO plan or the bundled plan?
Question 780 mispriced asset, NPV, DDM, market efficiency, no explanation
A company advertises an investment costing $1,000 which they say is under priced. They say that it has an expected total return of 15% pa, but a required return of only 10% pa. Of the 15% pa total expected return, the dividend yield is expected to be 4% pa and the capital yield 11% pa. Assume that the company's statements are correct.
What is the NPV of buying the investment if the 15% total return lasts for the next 100 years (t=0 to 100), then reverts to 10% after that time? Also, what is the NPV of the investment if the 15% return lasts forever?
In both cases, assume that the required return of 10% remains constant, the dividends can only be re-invested at 10% pa and all returns are given as effective annual rates. The answer choices below are given in the same order (15% for 100 years, and 15% forever):
You're considering a business project which costs $11m now and is expected to pay a single cash flow of $11m in one year. So you pay $11m now, then one year later you receive $11m.
Assume that the initial $11m cost is funded using the your firm's existing cash so no new equity or debt will be raised. The cost of capital is 10% pa.
Which of the following statements about the net present value (NPV), internal rate of return (IRR) and payback period is NOT correct?
Question 915 price gains and returns over time, IRR, NPV, income and capital returns, effective return
For a share price to double over 7 years, what must its capital return be as an effective annual rate?
Question 935 real estate, NPV, perpetuity with growth, multi stage growth model, DDM
You're thinking of buying an investment property that costs $1,000,000. The property's rent revenue over the next year is expected to be $50,000 pa and rent expenses are $20,000 pa, so net rent cash flow is $30,000. Assume that net rent is paid annually in arrears, so this next expected net rent cash flow of $30,000 is paid one year from now.
The year after, net rent is expected to fall by 2% pa. So net rent at year 2 is expected to be $29,400 (=30,000*(1-0.02)^1).
The year after that, net rent is expected to rise by 1% pa. So net rent at year 3 is expected to be $29,694 (=30,000*(1-0.02)^1*(1+0.01)^1).
From year 3 onwards, net rent is expected to rise at 2.5% pa forever. So net rent at year 4 is expected to be $30,436.35 (=30,000*(1-0.02)^1*(1+0.01)^1*(1+0.025)^1).
Assume that the total required return on your investment property is 6% pa. Ignore taxes. All returns are given as effective annual rates.
What is the net present value (NPV) of buying the investment property?
The present value of an annuity of 3 annual payments of $5,000 in arrears (at the end of each year) is $12,434.26 when interest rates are 10% pa compounding annually.
If the same amount of $12,434.26 is put in the bank at the same interest rate of 10% pa compounded annually and the same cash flow of $5,000 is withdrawn at the end of every year, how much money will be in the bank in 3 years, just after that third $5,000 payment is withdrawn?
The required return of a building project is 10%, given as an effective annual rate. Assume that the cash flows shown in the table are paid all at once at the given point in time.
The building firm is just about to start the project and the client has signed the contract. Initially the firm will pay $100 to the sub-contractors to carry out the work and then will receive an $11 payment from the client in one year and $121 when the project is finished in 2 years. Ignore credit risk.
But the building company is considering selling the project to a competitor at different points in time and is pondering the minimum price that they should sell it for.
| Project Cash Flows | |
| Time (yrs) | Cash flow ($) |
| 0 | -100 |
| 1 | 11 |
| 2 | 121 |
Which of the below statements is NOT correct? The project is worth:
Below is a screenshot from ASIC’s MoneySmart future value compound interest calculator website.
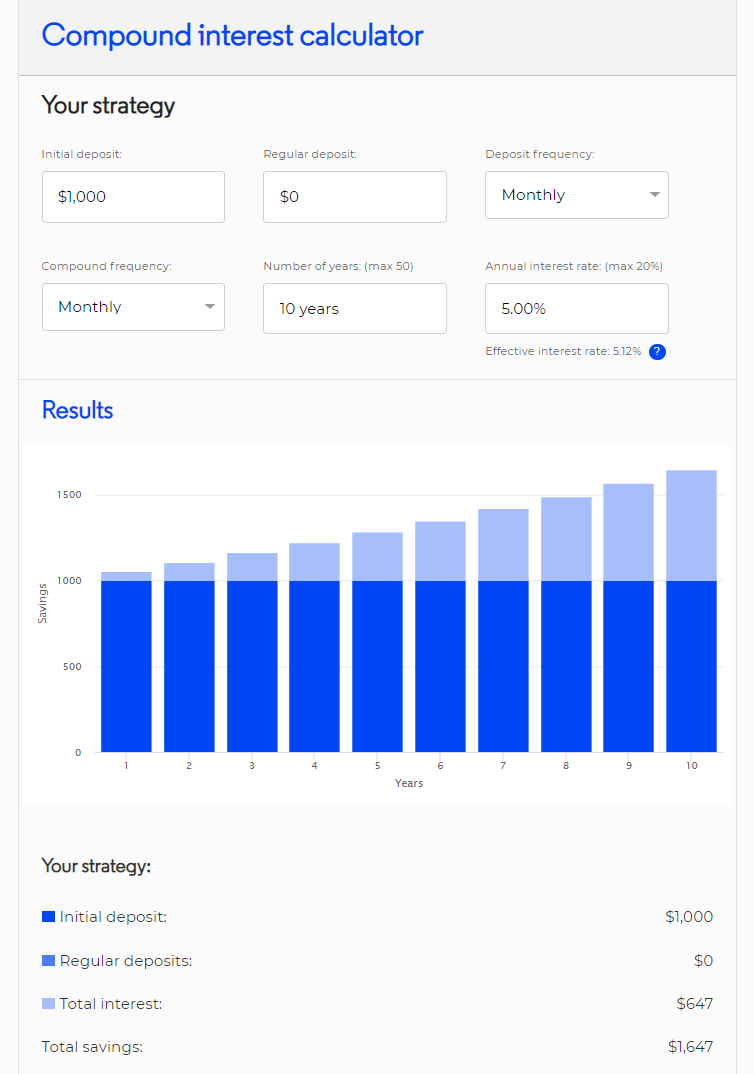
It shows that $1,000 invested in a bank account at 5% pa compounding monthly for 10 years will be worth $1,647, rounded to the nearest dollar.
Which of the following formulas replicates this result?
Below is a screenshot from ASIC’s MoneySmart future value compound interest calculator website.
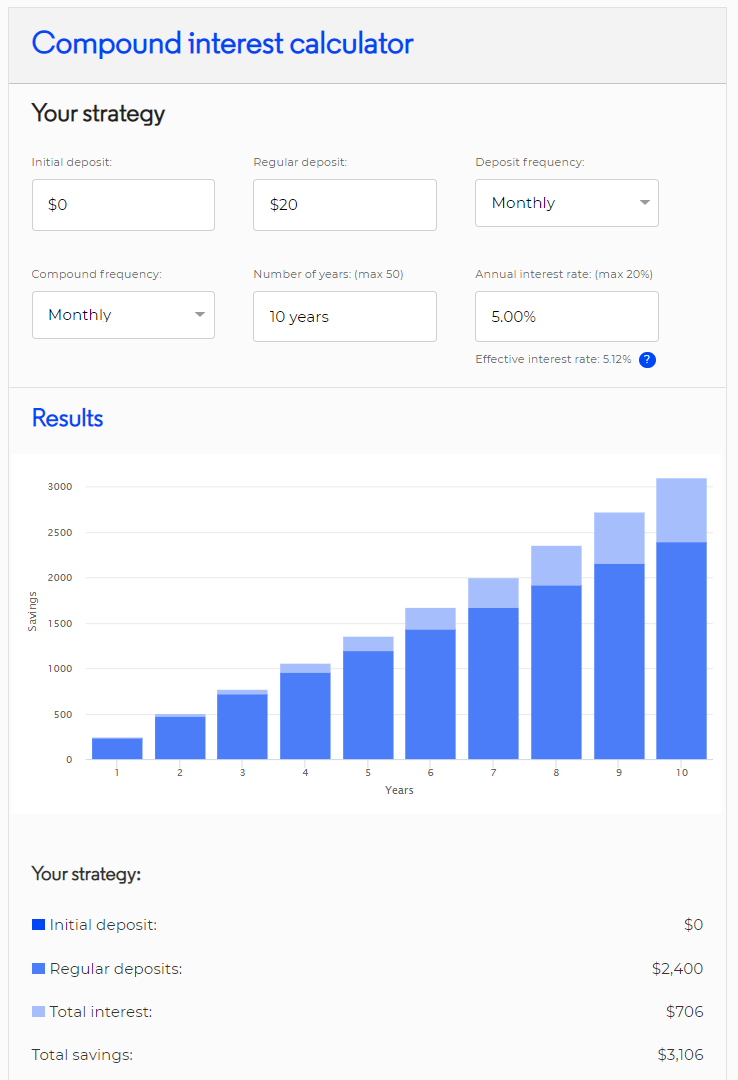
It shows that $20 invested (or deposited) into a bank account at the end of every month for 10 years at 5% pa compounding monthly will be worth $3,106 at the end of the 10 years, rounded to the nearest dollar.
Which of the following formulas replicates this result?
Your 18 year old friend is considering what to do with their working life until they retire at age 65. They've sought your advice.
For simplicity, ignore taxes and assume that wages will be paid annually in arrears and will be constant (zero growth). The abbreviation 'k' (Greek kilo) means thousands, so 1k is 1000.
Let the present be time zero (t=0) and the year that you retire be time 47 (t=47).
Your friend is deciding between working as a:
- Builder's apprentice for 2 years earning $20k pa (2 payments from t=1 to t=2 inclusive), then beginning work as a builder for $90k pa (45 annual payments from t=3 to 47 inclusive);
- Retail shop salesperson for $50k pa (47 payments from t=1 to t=47 inclusive).
You estimate that the required return is 5% pa with either career, and that they're equally risky. The cashflows are shown below:
| Career Choices and Cash Flows | ||
| Time | Builder | Retailer |
| 0 | 0 | 0 |
| 1 | 20 | 50 |
| 2 | 20 | 50 |
| 3 | 90 | 50 |
| 4 | 90 | 50 |
| ... | ... | ... |
| 47 | 90 | 50 |
Which of the following statements is NOT correct? Comparing the two alternatives, being a builder compared to a retail salesperson, the:
Your 18 year old friend is considering what to do with their working life until they retire at age 65. They've sought your advice.
For simplicity, ignore taxes and assume that wages will be paid annually in arrears and will be constant (zero growth). The abbreviation 'k' (Greek kilo) means thousands, so 1k is 1000.
Let the present be time zero (t=0) and the year of retirement is time 47 (t=47).
Your friend is deciding between:
- Studying at university for 3 years, costing $30k at the start of each year (3 cash outflows at t=0, 1 & 2), then beginning work as a financial planner for $100k pa (44 annual payments from t=4 to 47 inclusive);
- Working as a builder's apprentice for 2 years, earning $20k pa (2 cash inflows at t=1 and t=2), then beginning work as a builder for $90k pa (45 annual payments from t=3 to 47 inclusive);
You estimate that the required return is 5% pa with either career, and that they're equally risky. The cashflows are shown below:
| Career Choices and Cash Flows | ||
| Time | Planner | Builder |
| 0 | -30 | 0 |
| 1 | -30 | 20 |
| 2 | -30 | 20 |
| 3 | 0 | 90 |
| 4 | 100 | 90 |
| ... | ... | ... |
| 47 | 100 | 90 |
Which of the following statements is NOT correct? Comparing the two alternatives, being a financial planner compared to a builder, the:
It's the first day of the year and you currently have $4,500 in the bank. You plan to deposit $200 at the end of every year for the next 57 years, with the first payment made 1 year from now (from t=1 to t=57 inclusive).
If bank interest rates are 8% pa, how much money will be in your bank account a moment after making your last deposit in 57 years?
Find the net present value (NPV) of a mining project with the following cash flows:
- -$3,000 at time zero (t=0). This is the initial cost to prepare and build the mine and facilities. This cost is all paid upfront, though the builders will take 4 years to finish construction.
- $650 paid annually 45 times, where the first payment is made 5 years from now. So there are 45 annual payments from t=5 to t=49 inclusive. These are the positive cash flows from running the mine once it's built.
- -$1,400 at t=49. This is the clean-up cost of burying the mine and replanting native vegetation at the end of the project. This cost is paid at the same time as the last positive cash flow is received from running the mine.
The required return is 15% pa. All dollar figures above and below are in millions. The NPV of this project is:
You're considering starting a software company with an initial (t=0) cost of $71.
The first positive cash flow will be $10 in one year (t=1), and will grow by 2% pa for 3 years. So the next cash flows will be:
$10 at t=1;
$10.2 (=10*(1+0.02)^1) at t=2;
$10.404 (=10*(1+0.02)^2) at t=3;
$10.6121 (=10*(1+0.02)^3) at t=4.
From t=4 onwards, these positive cash flows will grow at the lower rate -3% pa (note the negative sign) in perpetuity. So the subsequent cash flows will be:
$10.2937 (=10*(1+0.02)^3*(1-0.03)^1) at t=5;
$9.9849 (=10*(1+0.02)^3*(1-0.03)^2) at t=6;
$9.6854 (=10*(1+0.02)^3*(1-0.03)^3) at t=7, and so on forever.
The required return is 10% pa. What is the net present value (NPV) of starting this company? All results above are rounded to 4 decimal points, and answer options below to 2 decimal points. The NPV of starting this company is:
Question 1091 NPV, perpetuity with growth, IRR, mutually exclusive projects, real estate
An investor owns an empty block of land that was bought for $3 million a few years ago, but could be sold at auction for $2 million now. The land has local government approval to be developed into either:
- Low-rise townhouses costing $11 million now (t=0) that can be rented for $2 million in the first year, paid at the end of that year (t=1), and then rent is expected to grow by 4% pa every year forever; or
- High rise apartments costing $90 million now (t=0) that can be rented for $14 million in the first year, paid at the end of that year (t=1), and then rent is expected to grow by 1% pa every year forever.
The government will only allow a single development so the projects are mutually exclusive.
These projects have the same risk and 9% pa required return. Both will be fully constructed in one year, at which point tenants will move in and pay rent annually in advance, with the growth rates given. Ignore all maintenance costs, tenant vacancies, taxes and so on. All answer options are rounded to 6 decimal places. Compare the two projects against selling the land. Which of the following statements is NOT correct?
Your boss the chief financial officer (CFO) asked you to complete the analysis of 3 different projects under consideration by the company's board. All projects require an initial investment and then provide a perpetuity of cash flows with zero growth. All are equally risky with the same 10% pa required return. All figures are rounded to 4 decimal places. The projects can all be accepted and funded, they're not mutually exclusive.
The projects' initial costs and perpetual annual cash flows were provided by the engineering and marketing departments, and the CFO completed some of the NPV's and IRR's but ran out of time and gave it to you to finish. This data is believed to be accurate. You calculated the remaining NPV's and IRR's in bold, and made some conclusions about which projects to accept or reject (stated in answer option d). The CFO thanked you for your swift work, but said there's just one thing wrong with your calculations or conclusions, and asked you to fix it up before the table and conclusions are shown at the board meeting tomorrow.
| Projects with 10% pa required return | ||||
| Initial cash flow at t=0 |
Perpetual annual cash flow from t=1 |
NPV | IRR | |
| ($m) | ($m) | ($m) | (% pa) | |
| Project A | -160 | 19 | 30 | (a) 11.875 |
| Project B | -2,200 | 190 | (b) -300 | 8.6364 |
| Project C | -20,000 | 1,600 | (c) -4,000 | 8 |
Which one of the following calculations or conclusions is NOT correct?