Your friend just bought a house for $400,000. He financed it using a $320,000 mortgage loan and a deposit of $80,000.
In the context of residential housing and mortgages, the 'equity' tied up in the value of a person's house is the value of the house less the value of the mortgage. So the initial equity your friend has in his house is $80,000. Let this amount be E, let the value of the mortgage be D and the value of the house be V. So V=D+E.
If house prices suddenly fall by 10%, what would be your friend's percentage change in equity (E)? Assume that the value of the mortgage is unchanged and that no income (rent) was received from the house during the short time over which house prices fell.
Remember:
r0→1=p1−p0+c1p0
where r0−1 is the return (percentage change) of an asset with price p0 initially, p1 one period later, and paying a cash flow of c1 at time t=1.
Question 210 real estate, inflation, real and nominal returns and cash flows, income and capital returns
Assume that the Gordon Growth Model (same as the dividend discount model or perpetuity with growth formula) is an appropriate method to value real estate.
An old rule of thumb in the real estate industry is that properties should yield a 5% pa rental return. Some investors also regard property to be as risky as the stock market, therefore property is thought to have a required total return of 9% pa which is the average total return on the stock market including dividends.
Assume that all returns are effective annual rates and they are nominal (not reduced by inflation). Inflation is expected to be 2% pa.
You're considering purchasing an investment property which has a rental yield of 5% pa and you expect it to have the same risk as the stock market. Select the most correct statement about this property.
You own an apartment which you rent out as an investment property.
What is the price of the apartment using discounted cash flow (DCF, same as NPV) valuation?
Assume that:
- You just signed a contract to rent the apartment out to a tenant for the next 12 months at $2,000 per month, payable in advance (at the start of the month, t=0). The tenant is just about to pay you the first $2,000 payment.
- The contract states that monthly rental payments are fixed for 12 months. After the contract ends, you plan to sign another contract but with rental payment increases of 3%. You intend to do this every year.
So rental payments will increase at the start of the 13th month (t=12) to be $2,060 (=2,000(1+0.03)), and then they will be constant for the next 12 months.
Rental payments will increase again at the start of the 25th month (t=24) to be $2,121.80 (=2,000(1+0.03)2), and then they will be constant for the next 12 months until the next year, and so on. - The required return of the apartment is 8.732% pa, given as an effective annual rate.
- Ignore all taxes, maintenance, real estate agent, council and strata fees, periods of vacancy and other costs. Assume that the apartment will last forever and so will the rental payments.
Your friend just bought a house for $1,000,000. He financed it using a $900,000 mortgage loan and a deposit of $100,000.
In the context of residential housing and mortgages, the 'equity' or 'net wealth' tied up in a house is the value of the house less the value of the mortgage loan. Assuming that your friend's only asset is his house, his net wealth is $100,000.
If house prices suddenly fall by 15%, what would be your friend's percentage change in net wealth?
Assume that:
- No income (rent) was received from the house during the short time over which house prices fell.
- Your friend will not declare bankruptcy, he will always pay off his debts.
Two years ago Fred bought a house for $300,000.
Now it's worth $500,000, based on recent similar sales in the area.
Fred's residential property has an expected total return of 8% pa.
He rents his house out for $2,000 per month, paid in advance. Every 12 months he plans to increase the rental payments.
The present value of 12 months of rental payments is $23,173.86.
The future value of 12 months of rental payments one year ahead is $25,027.77.
What is the expected annual growth rate of the rental payments? In other words, by what percentage increase will Fred have to raise the monthly rent by each year to sustain the expected annual total return of 8%?
Question 353 income and capital returns, inflation, real and nominal returns and cash flows, real estate
A residential investment property has an expected nominal total return of 6% pa and nominal capital return of 3% pa.
Inflation is expected to be 2% pa. All rates are given as effective annual rates.
What are the property's expected real total, capital and income returns? The answer choices below are given in the same order.
Three years ago Frederika bought a house for $400,000.
Now it's worth $600,000, based on recent similar sales in the area.
Frederika's residential property has an expected total return of 7% pa.
She rents her house out for $2,500 per month, paid in advance. Every 12 months she plans to increase the rental payments.
The present value of 12 months of rental payments is $29,089.48.
The future value of 12 months of rental payments one year ahead is $31,125.74.
What is the expected annual capital yield of the property?
Question 363 income and capital returns, inflation, real and nominal returns and cash flows, real estate
A residential investment property has an expected nominal total return of 8% pa and nominal capital return of 3% pa.
Inflation is expected to be 2% pa. All rates are given as effective annual rates.
What are the property's expected real total, capital and income returns? The answer choices below are given in the same order.
One and a half years ago Frank bought a house for $600,000. Now it's worth only $500,000, based on recent similar sales in the area.
The expected total return on Frank's residential property is 7% pa.
He rents his house out for $1,600 per month, paid in advance. Every 12 months he plans to increase the rental payments.
The present value of 12 months of rental payments is $18,617.27.
The future value of 12 months of rental payments one year in the future is $19,920.48.
What is the expected annual rental yield of the property? Ignore the costs of renting such as maintenance, real estate agent fees and so on.
Question 408 leverage, portfolio beta, portfolio risk, real estate, CAPM
You just bought a house worth $1,000,000. You financed it with an $800,000 mortgage loan and a deposit of $200,000.
You estimate that:
- The house has a beta of 1;
- The mortgage loan has a beta of 0.2.
What is the beta of the equity (the $200,000 deposit) that you have in your house?
Also, if the risk free rate is 5% pa and the market portfolio's return is 10% pa, what is the expected return on equity in your house? Ignore taxes, assume that all cash flows (interest payments and rent) were paid and received at the end of the year, and all rates are effective annual rates.
Question 415 income and capital returns, real estate, no explanation
You just bought a residential apartment as an investment property for $500,000.
You intend to rent it out to tenants. They are ready to move in, they would just like to know how much the monthly rental payments will be, then they will sign a twelve-month lease.
You require a total return of 8% pa and a rental yield of 5% pa.
What would the monthly paid-in-advance rental payments have to be this year to receive that 5% annual rental yield?
Also, if monthly rental payments can be increased each year when a new lease agreement is signed, by how much must you increase rents per year to realise the 8% pa total return on the property?
Ignore all taxes and the costs of renting such as maintenance costs, real estate agent fees, utilities and so on. Assume that there will be no periods of vacancy and that tenants will promptly pay the rental prices you charge.
Note that the first rental payment will be received at t=0. The first lease agreement specifies the first 12 equal payments from t=0 to 11. The next lease agreement can have a rental increase, so the next twelve equal payments from t=12 to 23 can be higher than previously, and so on forever.
Question 416 real estate, market efficiency, income and capital returns, DDM, CAPM
A residential real estate investor believes that house prices will grow at a rate of 5% pa and that rents will grow by 2% pa forever.
All rates are given as nominal effective annual returns. Assume that:
- His forecast is true.
- Real estate is and always will be fairly priced and the capital asset pricing model (CAPM) is true.
- Ignore all costs such as taxes, agent fees, maintenance and so on.
- All rental income cash flow is paid out to the owner, so there is no re-investment and therefore no additions or improvements made to the property.
- The non-monetary benefits of owning real estate and renting remain constant.
Which one of the following statements is NOT correct? Over time:
What type of present value equation is best suited to value a residential house investment property that is expected to pay constant rental payments forever? Note that 'constant' has the same meaning as 'level' in this context.
Question 522 income and capital returns, real and nominal returns and cash flows, inflation, real estate
A residential investment property has an expected nominal total return of 6% pa and nominal capital return of 2.5% pa. Inflation is expected to be 2.5% pa.
All of the above are effective nominal rates and investors believe that they will stay the same in perpetuity.
What are the property's expected real total, capital and income returns?
The answer choices below are given in the same order.
Question 529 DDM, real and nominal returns and cash flows, inflation, real estate, no explanation
If housing rents are constrained from growing more than the maximum target inflation rate, and houses can be priced as a perpetuity of growing net rental cash flows, then what is the implication for house prices, all things remaining equal? Select the most correct answer.
Background: Since 1990, many central banks across the world have become 'inflation targeters'. They have adopted a policy of trying to keep inflation in a predictable narrow range, with the hope of encouraging long-term lending to fund more investment and maintain higher GDP growth.
Australia's central bank, the Reserve Bank of Australia (RBA), has specifically stated their inflation target range is between 2 and 3% pa.
Some Australian residential property market commentators suggest that because rental costs comprise a large part of the Australian consumer price index (CPI), rent costs across the nation cannot significantly exceed the maximum inflation target range of 3% pa without the prices of other goods growing by less than the target range for long periods, which is unlikely.
One year ago you bought a $1,000,000 house partly funded using a mortgage loan. The loan size was $800,000 and the other $200,000 was your wealth or 'equity' in the house asset.
The interest rate on the home loan was 4% pa.
Over the year, the house produced a net rental yield of 2% pa and a capital gain of 2.5% pa.
Assuming that all cash flows (interest payments and net rental payments) were paid and received at the end of the year, and all rates are given as effective annual rates, what was the total return on your wealth over the past year?
Hint: Remember that wealth in this context is your equity (E) in the house asset (V = D+E) which is funded by the loan (D) and your deposit or equity (E).
Question 935 real estate, NPV, perpetuity with growth, multi stage growth model, DDM
You're thinking of buying an investment property that costs $1,000,000. The property's rent revenue over the next year is expected to be $50,000 pa and rent expenses are $20,000 pa, so net rent cash flow is $30,000. Assume that net rent is paid annually in arrears, so this next expected net rent cash flow of $30,000 is paid one year from now.
The year after, net rent is expected to fall by 2% pa. So net rent at year 2 is expected to be $29,400 (=30,000*(1-0.02)^1).
The year after that, net rent is expected to rise by 1% pa. So net rent at year 3 is expected to be $29,694 (=30,000*(1-0.02)^1*(1+0.01)^1).
From year 3 onwards, net rent is expected to rise at 2.5% pa forever. So net rent at year 4 is expected to be $30,436.35 (=30,000*(1-0.02)^1*(1+0.01)^1*(1+0.025)^1).
Assume that the total required return on your investment property is 6% pa. Ignore taxes. All returns are given as effective annual rates.
What is the net present value (NPV) of buying the investment property?
Question 941 negative gearing, leverage, capital structure, interest tax shield, real estate
Last year, two friends Lev and Nolev each bought similar investment properties for $1 million. Both earned net rents of $30,000 pa over the past year. They funded their purchases in different ways:
- Lev used $200,000 of his own money and borrowed $800,000 from the bank in the form of an interest-only loan with an interest rate of 5% pa.
- Nolev used $1,000,000 of his own money, he has no mortgage loan on his property.
Both Lev and Nolev also work in high-paying jobs and are subject personal marginal tax rates of 45%.
Which of the below statements about the past year is NOT correct?
Question 959 negative gearing, leverage, capital structure, interest tax shield, real estate
Last year, two friends Gear and Nogear invested in residential apartments. Each invested $1 million of their own money (their net wealth).
Apartments cost $1,000,000 last year and they earned net rents of $30,000 pa over the last year. Net rents are calculated as rent revenues less the costs of renting such as property maintenance, land tax and council rates. However, interest expense and personal income taxes are not deducted from net rents.
Gear and Nogear funded their purchases in different ways:
- Gear used $1,000,000 of her own money and borrowed $4,000,000 from the bank in the form of an interest-only loan with an interest rate of 5% pa to buy 5 apartments.
- Nogear used $1,000,000 of his own money to buy one apartment. He has no mortgage loan on his property.
Both Gear and Nogear also work in high-paying jobs and are subject personal marginal tax rates of 45%.
Which of the below statements about the past year is NOT correct?
| Major City Apartment Prices | |||
| One bedroom, one bathroom, around 55 square metre floor space, Dec 2018 | |||
| City | Advertised price | Currency | FX quote |
| London, Great Britain | 995,500 | GBP | 1.3 USD per GBP |
| Paris, France | 639,000 | EUR | 0.88 USD per EUR |
| San Francisco, USA | 859,000 | USD | 1 USD per USD |
| Shanghai, China | 6,300,000 | RMB | 6.9 RMB per USD |
| Sydney, Australia | 670,000 | AUD | 0.72 USD per AUD |
| Tokyo, Japan | 50,800,000 | JPY | 112 JPY per USD |
Which city has the most expensive apartment, measured in United States Dollars (USD)? Pay attention to the FX quotes.
Four retail business people compete in the same city. They are all exactly the same except that they have different ways of funding or leasing the shop real estate needed to run their retail business.
The two main assets that retail stores need are:
- Inventory typically worth $1 million which has a beta of 2, and;
- Shopfront real estate worth $1 million which has a beta of 1. Shops can be bought or leased.
Lease contract prices are fixed for the term of the lease and based on expectations of the future state of the economy. When leases end, a new lease contract is negotiated and the lease cost may be higher or lower depending on the state of the economy and demand and supply if the economy is:
- Booming, shop real estate is worth more and lease costs are higher.
- In recession, shop real estate is worth less and lease costs are low.
Which retail business person will have the LOWEST beta of equity (or net wealth)?
The saying 'safe as houses' suggests that house prices never fall. Examine the below graph of US house prices. Note that the vertical grey columns are periods when the US economy was in recession.
Did US house prices ever fall? US house prices have:
Question 1091 NPV, perpetuity with growth, IRR, mutually exclusive projects, real estate
An investor owns an empty block of land that was bought for $3 million a few years ago, but could be sold at auction for $2 million now. The land has local government approval to be developed into either:
- Low-rise townhouses costing $11 million now (t=0) that can be rented for $2 million in the first year, paid at the end of that year (t=1), and then rent is expected to grow by 4% pa every year forever; or
- High rise apartments costing $90 million now (t=0) that can be rented for $14 million in the first year, paid at the end of that year (t=1), and then rent is expected to grow by 1% pa every year forever.
The government will only allow a single development so the projects are mutually exclusive.
These projects have the same risk and 9% pa required return. Both will be fully constructed in one year, at which point tenants will move in and pay rent annually in advance, with the growth rates given. Ignore all maintenance costs, tenant vacancies, taxes and so on. All answer options are rounded to 6 decimal places. Compare the two projects against selling the land. Which of the following statements is NOT correct?
A house worth $700k is expected to earn $30k pa gross rent revenue and incur rental costs of $10k pa over the next year. Note that the units 'k' (Greek kilo) represent thousands. Assume that these cash flows are expected one year from now, so they’re received and paid annually in arrears.
If the gross rent revenue and costs increase by 2% per annum and houses can be valued as a perpetuity, what is the internal rate of return (IRR) of buying this house asset? Ignore taxes.
On 15 March 2024 journalist Tony Richardson wrote an interesting article “The ‘better way’ to build wealth than property investing” about Koda Capital financial adviser Sebastian Ferrando’s opinion that building wealth through levered equities is better than levered property. Their table and selected quotes are presented below:
Typical buy-to-let investors in Brisbane, Sydney or Melbourne will pay a deposit of 35 per cent and borrow 65 per cent from a lender…
The basic investment thesis is that buy-to-let investors can profit from leverage as they can make returns in the form of rent and capital growth on a $1 million asset after spending $350,000.
But Ferrando says anyone with savings beyond those needed to own a primary place of residence could also obtain leverage – known as a margin facility – on a share portfolio with a broker.
Take a hypothetical $1 million share portfolio with $500,000 committed by the investor and $500,000 committed by a broker on margin (accruing interest to be repaid). It could return 11.5 per cent a year after the interest paid on the margin debt and assuming an average return of 14 per cent per year, split across the Nasdaq and S&P 500. (Richardson, 2024)
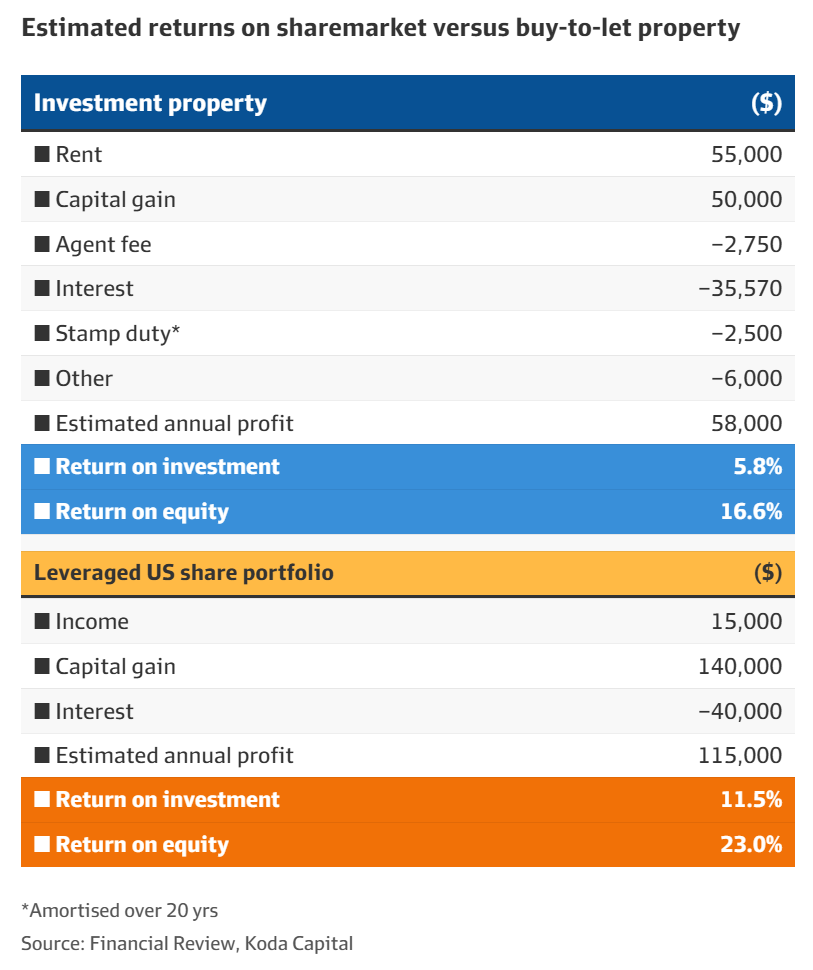
Assume a 4% pa risk free rate, 6% pa market risk premium (MRP), fairly priced property, stock and debt, ignore all taxes (except stamp duty) and currency differences, assume a single factor international CAPM. All figures are given to 6 decimal places.
Which of the following statements is NOT correct? The: